String Theory and the Geometry of the Universe’s Hidden Dimensions

Exploring the Hidden Dimensions of our Universe Through Geometry 
Shing-Tung Yau
Thursday, April 26, 2018 | 7:00pm 
UCI Student Center, Crystal Cove Auditorium 

Historically, advances in mathematics and our understanding of the physical universe have often gone hand in hand. Come hear from one of the world’s most distinguished mathematicians how this close interplay has continued to deepen in recent times with new mathematical breakthroughs in geometry and exciting physical theories that propose extra hidden dimensions in our universe.

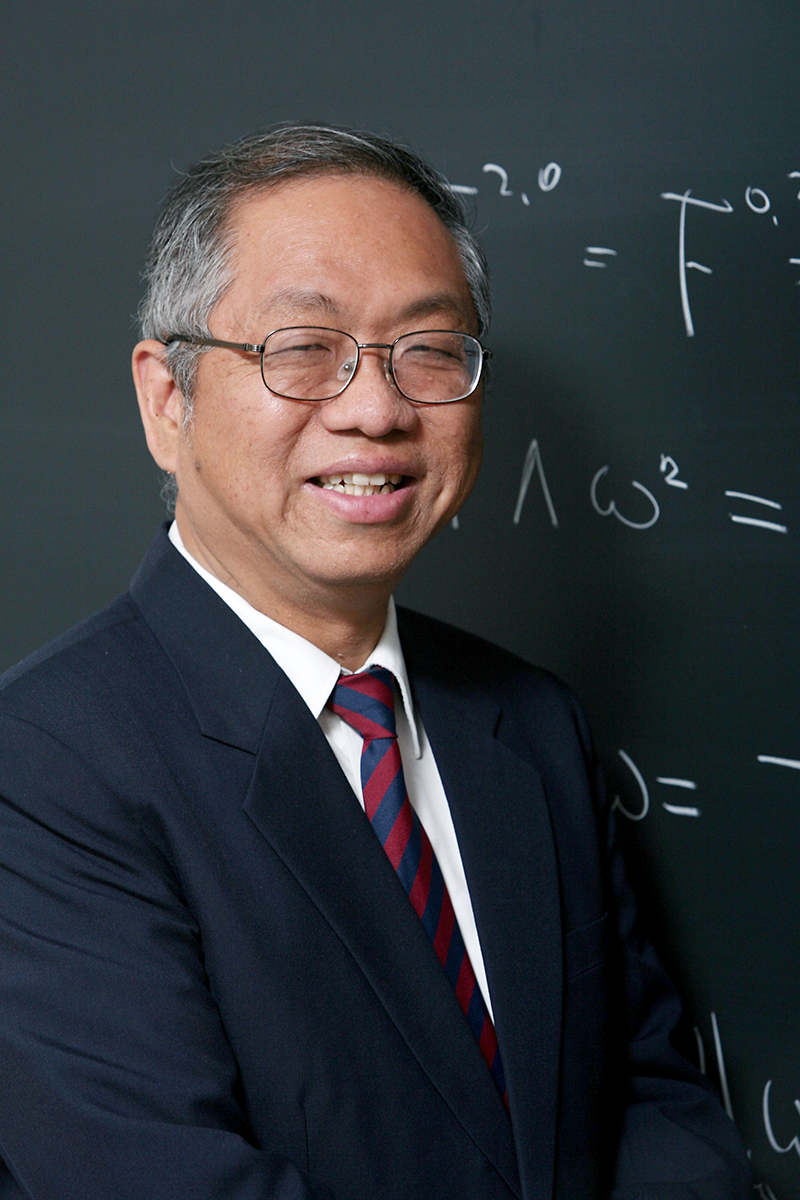Shing-Tung Yau is Harvard University’s William Caspar Graustein Chair Professor of Mathematics and Professor of Physics. His worldwide influence on mathematics and math/science education has few equals. He has made seminal contributions in many different fields of modern mathematics and also has had significant impact in physics, computer science, and technology. His many celebrated achievements include laying the mathematical foundation of Einstein’s general theory of relativity and many of today’s physical theories of spacetime with extra dimensions. Throughout his career, he has been a tireless educator having initiated a number of math and science competitions at the high school and university levels, established seven world-class mathematical research centers worldwide, and also wrote three noted popular science books. Dr. Yau was born in 1949 in Guangdong, China. He earned his Ph.D. from UC Berkeley in 1971, was appointed Professor at Stanford University in1974, and joined Harvard University in 1987. He is a member of the U.S. National Academy of Sciences, the American Academy of Arts and Sciences and the Academia Sinica. He has been awarded numerous top prizes including the Fields Medal, the MacArthur Fellowship, the Wolf Prize, and the U.S. National Medal of Science.

Please RSVP at https://ps.uci.edu/Yau

Parking for this event is available for $10 at the Student Center Parking Structure located on the corner of Pereira Dr. and West Peltason. This lecture is free and open to the public. School groups and media representatives should contact Tatiana Arizaga at tarizaga@uci.edu. 

Abstract: When numbers are added in the usual way, "carries" occur along
the way. Making math sense of the carries leads to all sorts of
corners, in particular to the mathematics of shuffling cards. I will
show that it takes seven ordinary riffle shuffles to mix up 52 cards and
explain connections to fractals and other lovely mathematical objects.
This is a talk for a general audience, no specialist knowledge needed.

Quantum cohomology is a deformation of the classical cohomology algebra of an algebraic variety X that takes into account enumerative geometry of rational curves in X. This has many remarkable properties for a general X, but becomes particularly structured and deep for special X. One of the most interesting class of varieties in this respect are the so-called equivariant symplectic resolutions. These include, for example, cotangent bundles to compact homogeneous varieties, as well as Hilbert schemes of points and more general instanton moduli spaces. A general vision for a connection between quantum cohomology of sympletic resolutions and quantum integrable systems recently emerged in supersymmetric gauge theories, in particular in the work of Nekrasov and Shatashvili. In my lecture, which will be based on joint work with Davesh Maulik, I will explain a construction of certain solutions of Yang-Baxter equation associated to symplectic resolutions as above. The associated quantum integrable system will be identified with the quantum cohomology of X. If time permits, we will also explore K-theoretic generalization of this theory.